Suppose we have two lenses of focal lengths f1 and f2 separated by a distance x as shown in fig below.
L1, L2 correspond to the position of two lenses while the transverse planes through L1 and L2 will serve as input and output planes respectively. AB is the incident ray and CD is the emergent ray.

We know, there is translation of the rays of the rays of light from the input to the output plane, so we can write the system matrix for this co-axial system of lenses as

Where [Sthin]2 and [Sthin]1 are system matrices for second and first thin lenses respectively. Tm is the translation matrix within the optical system.






This is the system matrix for the combination of two lenses.
If the medium on two sides of the optical system be same (ni=n0), then the focal length of the system is given as:

where c is the element of the system matrix
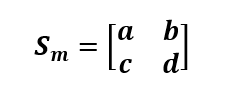
So, we have the focal length of the combination of two lenses as

where f is called the focal length of equivalent lens system.
Also from the equation (2), we have

If lenses are in contact, x=0
Therefore focal length of the combination of two lenses in contact is given by

This equation (4) also called the combination of lenses formula.
